Tartini tones and temperament: an introduction for musicians

Where do you hear Tartini tones?

Imagine that, in a loud, sustained chord, senza vib, your neighbour is playing a sustained A5 at 880 Hz, or 880 vibrations per second (A5 is an octave above A4, the tuning A at 440 Hz) . You have the third above (C#6), and let's say you are playing that as an equal tempered third, at 1109 Hz. Both of you hear an annoying, buzzy low note, whose frequency turns out to be the difference between those of your notes: 229 Hz in this case. This is the Tartini tone or terzo suono, named for the violinist/composer/theorist who wrote on it. I still remember my surprise on hearing it for the first time. Where did that low note suddenly come from? It seemed to be inside my head! Later I discovered that indeed it was.

In the example above, it's an ugly surprise, because 229 Hz is not in your chord: it's 66 cents or two thirds of a semitone sharper than A3 (220 Hz) – it's closer to A# and than A. The natural reaction is to play the C# a little flatter: if you pull it from 1109 down to 1100 Hz, the Tartini tone falls to 220 Hz, so it's now in tune and no longer annoys you. The effect is spectacular: you've lowered your note by 9 Hz, which is 14 cents at 1100 Hz, so the Tartini tone has come down by 9 Hz, but at 220 Hz, a 9 Hz change is 67 cents—two thirds of a semitone.

You've pushed your interloping note into tune; even better, your A-C# chord now sounds particularly pure. This slightly narrower third is a pure third, or a third in just intonation: it has a frequency ratio of 1100/880 or 5/4. This ratio has a long history in harmony and temperament. It has some physical importance, too.

About harmonics

Suppose two cellists tune their A strings in exact unison at 220 Hz. One of them then plays what string players call a 'natural harmonic': touching the string very lightly, but not stopping it. If she touches the string (with art and cunning) at a position 1/n along its length, n a whole number, then it can vibrate in n loops, with (n−1) stationary points along its length, not counting nut and bridge. The most common one is the touch fourth, illustrated below. 


The touch fourth. Touch the string at one fourth of its length (which also happens to be the position where stopping raises the string's note by a fourth, hence the name) and it produces a wave that is four times shorter than the open string, its frequency 4 times higher and its pitch two octaves higher. In the sketch, the vertical dimension is exaggerated.
If one cellist plays a touch fourth, that note is almost exactly two octaves higher, an A5 at 4*220 Hz = 880 Hz. Now the other cellist touches the string one fifth of the way along (a touch major third, because it's above the position to stop the string for that interval). This produces a frequency of 5*220 Hz = 1100 Hz, a C#5 in just intonation.

In fact, when any bowed string or wind instrument plays A2, it will usually produce a sound spectrum that contains a collection of harmonics, including the fourth and fifth, at 880 and 1100 Hz. So, in this sense, our ears are very accustomed to just thirds.

Where does the Tartini tone come from?

We can easily identify two contributing causes. One is a mechanical response in a part of the inner ear called the basilar membrane, a remarkable structure whose mechanical properties contribute to our sense of pitch. (More about pitch perception here.) These properties are non-linear, meaning that twice as much force does not produce twice as much deformation. A few lines of maths show that a non-linear function of two vibrations at different frequencies produces a component at the difference frequency — the Tartini tone. (Also other components, called heterodyne components). This effect is stronger for large vibrations, which is why we notice Tartini tones especially in loud playing of reasonably high notes, when you are close to the instruments. The pattern of nerve impulses produced by the two vibrations also contributes to the effect.

Temperaments

I mentioned temperament above, because the just thirds that are required to put the Tartini tone in tune create, on keyboards at least, the problem that temperament aims to solve. Suppose we make C-E a just third, with ratio 5/4. Then set E-G# at 5/4, and G#/B# at 5/4, too. The interval between C and the B# above is now (5/4)³ = 1.95. This is a long way flat from an octave, which is a ratio of 2. This is fine if you play a string or wind instrument: even if you use the same fingering for B# and C, you simply adjust finger position or lip force to play in tune. For the harpsichord and organ, however, these options are not available, and a number of temperaments exist to minimise the unpleasantness caused by setting B# = C, F# = Gb etc. A huge literature exists to describe and to compare them.

Equal temperament (ET) is one compromise: it uses equal thirds and equal semitones (whose ratios are respectively 2^1/3 (major), 2^1/4 (minor) and 2^1/12). The intonation problems are thus spread equally over all intervals, one of whose consequences is that the only difference in the sound of different keys is the difference in pitch. So Db major sounds a semitone higher than C major, but the intervals have the same ratios. (Some composers complain about this: they want Db to be sadder than C. Experienced amateur players like me, however, can assure them that the sadness will be provided by the extra wrong notes that gratuitous writing in five flats is likely to produce!)

Equal temperament does, however, produce the impure thirds and out-of-tune Tartini tones like the one we discussed above.

Equal temperament and the piano

So why does this vexed problem for harpsichordists not affect pianists? There are two reasons. First, harpsichordists can minimise the problem by putting the pieces in flat keys before the interval, during which they retune for the sharp key pieces in the second half. For a piano, this is impossible, so there's no point worrying about it. The second, more important reason has to do with the triplets or duplets of strings on the piano.

Good piano tuners tune the strings in a duplet or triplet to slightly different frequencies. This has three important effects. First, it means that, soon after being struck, the strings are not vibrating in phase: while one string is going down, its neighbours in the triplet are going up. This reduces the vibrational force on the bridge, which in turn means that the strings lose their energy less quickly. This gives the piano its long sustain after-sound. Second, the slight differences give the sound an interesting liveliness, somewhat like the chorus effect that distinguishes the sound of a violin section from that of one violin. Third, it makes it impossible to distinguish Tartini tones, and reduces the unpleasantness of impure intervals.

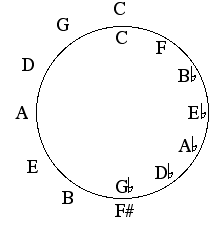
Fourths, fifths and the 'circle' At first, it seems that fourths and fifths don't cause the same problems. A pure fifth has the ratio 3/2 = 1.500 and a fourth 4/3 = 1.333, while the ET fifth and fourth are 27/12 = 1.498 and 25/12 = 1.335. So the differences are tiny. The trouble comes if we stack them up. Nearly every musician spends some time, first in wonder then in worry, on discovering the circle of fourths (or circle of fifths). Play four ascending notes in a major scale, say C-F. Then another: F-Bb. Continuing thus, and setting Gb = F#, we have C-F-Bb-Eb-Ab-Db-Gb(=F#)-B-E-A-D-G-C. On a piano, Gb = F# so, after 12 fourths, we have covered five octaves and come back 'home' to C — hence the circle, which is shown at right. Twelve pure fourths (4/3)12 = 31.6. On a keyboard, where Cb = B, 12 fourths is five octaves, which is 25 = 32.0. Out by 1.3% or a quarter of a semitone, called the Pythagorean comma — it's not a new problem! Not that we stack them up like this often, but see below.

Do it yourself

The Tartini tone is most easily heard between two notes that are fairly loud (i.e. close to you) and high. To demonstrate, I like to use two descant recorders, which I can blow simultaneously, fingering G on one (TXXXOOO), then successively B, C and D on the other (TXOOOOO, TOXOOOO, OXOOOO). (These fingerings produce C then E, F and G on an alto recorder.) Once you recognise Tartini tones, they can be helpful in chamber music for tuning thirds (as in the example above) and also fourths and fifths.

Heterodyne frequencies vs Tartini tones

In the Tartini tone examples discussed above, two tones of frequencies f₁ and f₂ produce the sensation of a pitch with frequency f₂−f₁ even though there is no sound wave with that frequency (and nothing outside your head is vibrating with frequency f₂−f₁). Two signals with frequencies f₁ and f₂ can however produce a signal with frequency f₂−f₁ (among others) if they are input to a nonlinear system, by a process called heterodyning or nonlinear superposition. (f₂−f₁ is a heterodyne frequency, as is f₂+f₁, 2f₁−f₂, and others.) The details are interesting and I introduce them here and give some examples.

Now a sounding woodwind reed or a brass instrument player's lips are examples of (strongly) non-linear systems. So playing the note with f₁ and singing the note with f₂ can produce a note with frequency f₂−f₁. This heterodyne tone is often much stronger than a Tartini tone. Further, in this case there really is air vibration at frequency f₂−f₁, as one can see in the spectrum of its microphone output. In my nomenclature, this distinguishes heterodyne components from pure Tartini tones, for which there is no acoustic signal at f₂−f₁.

Heterodyne production is used to produce notes below the range of an instrument. If you play a note with f₁ and sing the note a fifth higher (f₂ = 3f₁/2) then the difference frequency f₂−f₁= f₁/2: you hear the note one octave below the instrument's note. To go two octaves below, sing a just major third above (f₂ = 5f₁/4). This technique is very important (and very impressive) on the didjeridu.

A bowed string is also a nonlinear system. So, when two notes are played as double stops on a violin, heterodyne components are produced. If the two notes were played on two violins, the (weak) Tartini tone would be heard at f₂−f₁, but there would be no acoustic signal at this frequency. Played as a double stop on one violin, the (strong) heterodyne component at f₂−f₁ is not only heard, but is present in the sound signal, because the same bow and its stick-slip action is coupled to the motion of both strings. The heterodyne signal in this case is not an illusion, in the sense used above.

Because (pure) Tartini tones are weak, they are best heard between reasonably high notes, so that the Tartini tone falls in a range where the ear is sensitive. Heterodyne tones are strong and make impressive bass notes on didjeridu, saxophone or brass instruments.

More resources

• We give a more detailed introduction at Tartini tones, consonance and temperament.
• More about interference, and a large collection of sound files is at Interference beats and Tartini tones.
• Physclips Waves and Sound gives a broad, multimedia introduction to sound, and the relevant chapters hear are interference and Speech and Hearing.
• The circle of fourths/ fifths is so important in music theory that I've written an orchestral overture using the circle as a central harmonic and melodic component. See Circle of Fourths.
• See Notes and frequencies to convert notes, frequencies, MIDI numbers etc.
• Strings and standing waves gives a simple introduction to the vibrations in strings.

* I use the word 'illusion' to stress that, for example, when you hear pure tones at 200 Hz and 300 Hz together producing a Tartini tone with pitch corresponding to a pure tone at 100 Hz, there is no acoustic power at 100 Hz. It is true that three cycles of 300 Hz takes the same time as two cycles of 200 Hz, so the pattern repeats every 10 ms. What is interesting is that, from those two pure tones, your ear (or suitable software or hardware) can create the impression of a tone at 100 Hz. In a nice analogy that also involves non-existent frequency components, it's interesting to refer to the colour yellow, as produced on a computer monitor or television, as a visual illusion, in the sense that no yellow light is ever emitted from those screens. See this site for details.) If you would prefer to call a Tartini tone a real note (or computer yellow a real colour), that's fine.