
The paper below was published in Acoustics Australia, Vol 24, pp 11-15 (1996). For more recent publications, and an introduction to the physics of the instrument, see our main site:
Neville Fletcher
Research School of Physical Sciences
Australian National University
Canberra ACT 0200
| ABSTRACT: The didjeridu of the Australian aboriginal people is an ancient and deceptively simple instrument, consisting of a length cut from a narrow tree trunk or branch and hollowed by the successive action of fire and termites. Skilled players, however, are able to produce a wide repertoire of interesting musical effects including a rhythmic drone, striking timbre changes, and sounds that are voiced as well as played. This paper outlines the passive acoustics of the didjeridu tube, the active acoustics of the sound-production process, and the mechanisms by which the various musical effects are produced. |
To play the didjeridu, the musician seals the narrow end of the tube around his mouth, blows, and vibrates his lips under muscular tension in very much the same way as used in playing a brass instrument such as the tuba. The didjeridu uses air at rather a high rate so that, to playa sustained tone, the player adopts the technique of "circular breathing". After playing normally for a few seconds, he expands his cheeks with air, seals off his mouth from his throat with the back of the tongue and, while using the stored air to maintain the tone, takes a quick breath through his nose. This technique is common on certain other instruments, such as Indonesian flutes, and is now used routinely by oboists and even flute players to play without breath breaks for as long as several minutes. In these instruments, with their much smaller breath demand, the objective is to maintain an even tone and cover up any effect of the breathing. With the didjeridu, however, the player makes a virtue of necessity and emphasises the rhythmic breathing cycle to produce a pulsating drone. The pulsations are usually further decorated by tongue vibrations, so that the player effectively says unvoiced words such as "ritoru" or even "didjeridu", with the final "u" sound prolonged. The westernised name "didjeridu" for the instrument perhaps arises from this circumstance, though it may perhaps be a word from some aboriginal language, now extinct.
There has been only a little written about the acoustics of the didjeridu [1,2] or about its playing techniques [3,4]. The instrument itself, however, has become increasingly used in popular music by groups such as Gondwanaland, and was earlier made widely known on television through the efforts of Rolf Harris. A few simple calculations and measurements, however, allow us to understand a good deal about this interesting instrument.

If the flare is extremely small so that the horn is nearly cylindrical, then x1 becomes very large and tan-1knpi approaches pi/2. The resonance frequencies are then fn = (n - á)c/2L' which form the series of odd harmonics that we expect, for example as the playing frequencies of a clarinet, starting with a quarter of a wavelength equal to the tube length. More generally, if the flare is fairly small, we can expand the result (1) to arrive at the approximate expression
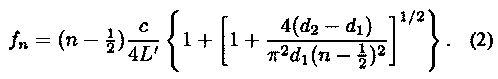
| Length L (cm) | 159 | 144 | 149 |
| Diameter d1 (mm) | 31 | 26 | 30 |
| Diameter d2 (mm) | 36 | 60 | 40 |
| Frequency f1 (Hz) | 60 | 80 | 64 |
| Drone pitch | B1 | E2 | C2 |
It is interesting to note that traditional makers and players seem to have little concern with either the drone frequency or the interval to the second mode - the first two instruments in the table are actually by the same maker. Indeed, a good player can produce most of the nuances of traditional performances on a piece of plastic pipe of appropriate diameter and length! When used in popular Western music, however, it is necessary to select a didjeridu of appropriate pitch to match the keyboard instruments, though in some multi-track recordings the didjeridu is actually recorded first and then pitch-shifted, the player having made some adjustment for the associated change in tempo. Breaking with tradition, Graham Wiggins has made the perhaps obvious extension of building a didjeridu with keys to open one or more holes near the foot and so allow the drone pitch to be changed.
The familiar reed valves of oboes and clarinets are of the ( - , + ) type, as also are the metal reeds used in organ reed-pipes. The human vocal folds are usually modelled as having the configuration ( +, + ), as are the vocal organs of birds (the syrinx), though the models used are generally more complex than this. The lips of players of brass instruments, such as the trumpet or tuba, and of the didjeridu, are either of configuration ( +, - ) or ( +, + ), and possibly change character between different playing regimes [7] . It is probably necessary to use a rather complex model for the vibrating lip valve, such as has been developed for the human vocal folds [8], but this has not yet been attempted. We must therefore be satisfied for the present with simpler models.
If we define the acoustic admittance of a pressure-controlled valve under blowing pressure, as viewed from the instrument, to be the ratio of the small-signal acoustic flow out of the instrument to the small-signal acoustic pressure in the instrument mouthpiece, then there is the possibility of self -sustained oscillation if the resistive part of this impedance (the acoustic conductance) is negative, to overcome the losses in the system, and if the reactive part can be balanced by the reactive admittance of the instrument tube and the players mouth, taken together. In all cases, the first condition requires that the blowing pressure should be greater than some threshold value determined by the tension of the lip muscles, which itself depends on the pitch of the note being played [9,10].
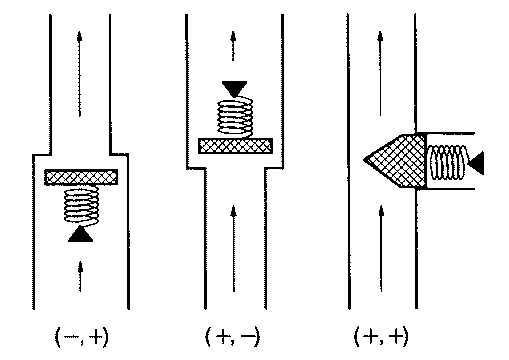
Figure 1. The three types of simple pressure-controlled valve. Air flow direction is shown with an arrow.
Provided a blowing pressure greater than this minimum is used, then the acoustic admittance of a lip-valve generator can be shown [9] to have a form like one of those shown in Fig. 2. For such a ( +, - ) or a ( +, + ) valve, the acoustic conductance - the real part of the admittance - is large and negative at a frequency either just above or just below the resonance frequency of the lip-valve, which is determined by lip mass and muscular tension. At other frequencies the conductance is relatively small and may be either positive or negative. The magnitude of this peak negative conductance is sufficiently large that it is able to overcome the positive conductance losses in the rest of the system and force it into oscillation. While this can happen over a considerable frequency range if the lip resonance frequency is adjusted - a skilled trombone player can play a glissando without moving the instrument slide-the oscillation is most easily sustained near an impedance maximum of the tube, where its positive conductance is least. The acoustic impedance of the player's mouth also plays an important role in sustaining the lip oscillation - a role that can be appreciated when we realise that it is possible to buzz the lips at their resonance frequency even in the absence of any instrument tube [10].
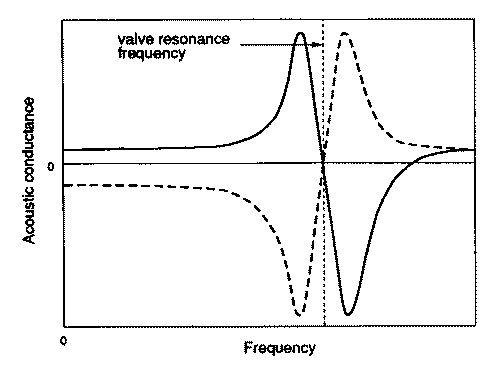
Figure 2. Acoustic conductance of a ( +, - ) valve (full line) and of a ( +, + ) valve (broken line). The resonance frequency for free vibration of the valve is shown.
This is the operating regime for a didjeridu-the player adjusts lip tension so that the lip resonance is close to the first tube resonance. To produce the second mode, the player must use a much higher lip tension to raise the lip resonance frequency, and this requires, in turn, a greater threshold blowing pressure. The actual pressures used are, of course, well above the threshold value. Measurements [1] show that a didjeridu player typically uses a pressure of about 1-2 kPa (10-20 cm water gauge pressure) for the drone note and about 4-5 kPa to produce the second mode. Some players can produce the third mode and even higher modes, but they are rarely used. Indeed, even the second mode is only employed for brief accents, and not as a sustained tone.
Because, unlike the reed valve in a clarinet, the lip valve operates at very nearly its resonance frequency [7,9,10], the motion of the player's lips is nearly sinusoidal. The average lip opening is determined by the blowing pressure, and the amplitude of the lip vibration is such that the lips just about close once in each cycle. If P0 is the steady blowing pressure, p the pressure just inside the mouthpiece of the instrument, and x = a0 + a sin 2pi ft the lip opening, then the volume flow U through the lip valve is
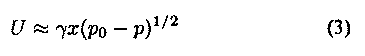
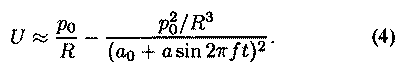
Clearly such a waveform has many harmonics, and this accounts for the rich sound of the didjeridu, and of lip-excited instruments in general. The relative strengths of the upper harmonics are not well predicted by this simple flow waveform, however, for several reasons. The flow waveform gives a spectral envelope which is initially nearly constant and then declines at about 12 dB/octave. The assumption that R is constant, however, is not very good, and this resistance is less for the upper harmonics than for the resonant fundamental, except for accidental near-coincidences with higher horn resonances. Finally, the transfer function between flow spectrum and acoustic radiation rises at 6 dB/octave at low frequencies and is then flat above about 3 kHz for the didjeridu horn. Despite these reservations, however, this simple treatment does give a fair idea of spectral behaviour.

Figure 3. The flow waveform through a lip-valve at several amplitude levels, as given by Equation (4).
Note that the sound spectrum of the didjeridu, as for all sustained-tone instruments (except when playing "multiphonics" or other special effects), is strictly harmonic. The fact that the upper modes of the pipe are not in harmonic relation to the fundamental affects only the strength of certain harmonics. If one of the upper pipe modes is sounded instead of the fundamental, then this sound will itself be accompanied by its own set of harmonics.
We should now consider the effect of the player's mouth cavity on sound quality. The player's lip opening varies nearly sinusoidally with time, as we have seen. The time spent at each opening is inversely proportional to the lip speed at that opening. If the lips just close each cycle so that a = a0, the fraction of time spent at opening x can then be shown to be proportional to [x(2a0 - x)]-1 which is sharply peaked at x = 0 and 2a0, so that the lips spend most of their time either nearly fully open or nearly closed. Seen from the instrument tube, therefore, the player's mouth is mostly either blocked off by the closed lips or else forms a Helmholtz resonator consisting of a closed volume vented by the lip opening. The resonance frequency of this resonator can be estimated from our experience with whistling, in which the whistle frequency is the resonance frequency of the same Helmholtz resonator. Since the lip opening is similar, within a factor of less than ten, in the two cases, the attainable resonance frequencies should be the same within about a factor three. We therefore expect that it should be possible to vary the resonator frequency over a range from about 500 Hz to about 3 kHz by changing the mouth volume with the tongue.
It is fairly easy to understand the effect of such a resonator on the lip-valve flow and hence on the radiated sound spectrum. The resonator is rather highly damped by the flow resistance through the lip valve so that its bandwidth encompasses the frequencies of several harmonics of the drone frequency. The acoustic flow through the lip valve will be enhanced for these harmonics, so that the acoustic spectrum will exhibit a "formant band" rather like those of the human voice and, indeed, arising from similar causes. Details are more complicated than this, of course, because the opening from the mouth to the instrument is changing with time. While the didjeridu can be played with a dull drone, lacking obvious formants, this is not usual for good players. Fig. 4 shows two examples of such formants, which play an important role in producing the characteristic sound of the didjeridu. In the first example, there is a pronounced formant band at about 1500 Hz, while in the second example the player has reduced the volume of his mouth so as to raise formant frequency to about 2.2 kHz. In each case there is some evidence for a lower vocal-tract formant at about 500 Hz. Because the frequency range of these formants is similar to that of human vowel formants, they have a similar aural effect. In normal playing, using circular breathing, these formants are produced in a rhythmic manner as the mouth volume changes, but they are often made a tonal feature of the performance.
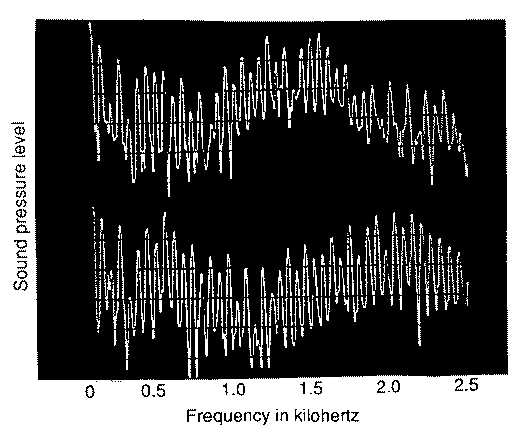
Figure 4. Formant bands in the didjeridu sound. In the upper trace, there is a mouth-cavity formant at about 1.5 kHz, while in the lower trace this has been shifted to about 2.2 kHz by constricting the mouth.
There is one other aspect of performance technique that deserves detailed acoustic comment. This is the use of vocal sounds to augment the drone of the didjeridu. Because of the acoustic coupling between the vocal folds in the throat and the player's vibrating lips, the interaction is quite complex. Suppose that the player's vocal folds vibrate at a frequency fV. Then this produces pulses of flow in the same way as described for the lip valve and illustrated in Fig. 3. The flow entering the mouth, and therefore the mouth pressure p0 of (4), thus contains all harmonics nfV of the vocal-fold frequency. When this flow is convolved with the nonlinear flow through the lips, which are vibrating with frequency fL, as in (4), the result is the production of all frequencies nfV ± mfL, those with greatest amplitude having small integer values (l or 2) for m and n.
The simplest example of this frequency mixing occurs when the player sings a steady tone at a frequency simply related to the drone frequency. A typical example is the singing of a note that is a just major tenth (frequency ratio 5/2) above the drone fundamental. The cross term fV - 2fL then has a frequency fL/2 and this is accompanied by all its harmonics from the other cross terms. The sound is therefore an octave below the original drone frequency. There is not be much radiated energy in this sub-octave fundamental, but the subjective pitch is generated strongly from the sequence of harmonics. Because of the low pitch and the strength of the higher harmonics, the sound has a rough rasping quality which is very effective. A rather similar result can be obtained by singing a note a perfect fifth (frequency ratio 3/2) above the drone fundamental.
Finally, we should remark that players of the instrument often use it to accompany traditional songs or stories and, to this end, embellish their playing by adding the sung sounds of barking dingos, brolgas and other animals. The pitch of these vocal sounds is rather high so that frequency mixing does not have such a pronounced effect, and the sounds can be made easily recognisable.
Fig. 5 shows a spectral display of a short passage of didjeridu playing. In this representation, time is along the horizontal axis and frequency on the vertical axis, with the density of shading indicating the sound pressure level. Two things are immediately obvious. The first is that the harmonic structure of the sound is clearly evident in the closely spaced dark bands running horizontally in the figure. The second feature is the formant bands, which show up as darker regions on the plot and vary with time. Articulation and circular breathing divide the time record into repeating segments. Features of this type will be familiar to anyone involved with human speech analysis.
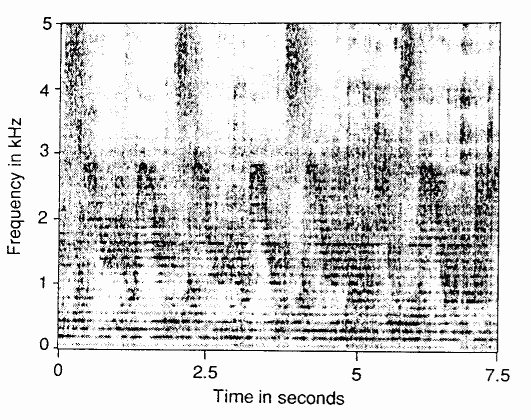
Figure 5. Time-frequency display of the sound of a didjeridu during a typical playing sequence. Note the harmonics of the drone frequency, the shifting formant bands, and the articulation noise.